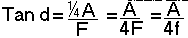John M. Pierce's HobbyGraph Articles

.

When d - angle of obliquity, A = diameter of the speculum, F = Focal length of the speculum, and f = ^F/_A. The maximum diameter of the distorted image is given by the formula a = ^11d/_f2 when a is the aberrational diameter of the image in seconds of arc, and d is given in minutes of arc.
      We will now find value of "a" for several "f" values.

	f8	Tan d = 1/32		d = 107'	a = 18.4"
	f12	Tan d = 1/48		d = 71'	a = 5.4"
	f16	Tan d = 1/64		d = 54'	a = 2.3"
	f20	Tan d = 1/80		d = 43'	a = 1.2"
	f24	Tan d = 1/96		d = 36'	a = 0.69"

the theoretical resolving power of a telescope in seconds of arc according to Dawes is given by the ratio 4.36/A. When the aberration in the image drops to the value it is no longer effective and such a mirror is capable of performing with the same efficiency as a centered mirror. The resolving power of a 4 inch mirror is 4/A or 1.14". Thus we see that a 4 inch Herschellian telescope of f20 focal ratio is capable of theoretical performance oa an instrument of this size having a centered mirror.
      Actually it is only at high powers that the theoretical extremes are possible, and so long as the image magnified by the eyepiece does not exceed the resolving power of the eye - about 4 minutes of arc - so no harm is done. This allows us to use a moderate focal length with ordinary magnifications.
      Practically, it is impossible to place the eyepiece as near the axis as we have assumed in our previous calculations, as the side of the observer's head would interfere. It must be about 1 1/2" farther out, which materially increases the angle of obliquity, demanding longer focal lengths or lower powers.
      Figure 2 shows a modification of the Herschellian or rather a combination of this with the Newtonian. This "Newtellian" type reduces the increased angle of obliquity by inserting a small diagonal just outside the cylinder of light falling on the mirror, to turn the eye position to the side. Incidentally this is a much more convenient position for the observer.
      We will investigate a telescope of this type in which the diagonal mirror is 1 1/2" inside the focal plane and the line of sight strikes the diagonal 1/2" outside the cylinder or rays of light from the object observed that falls on the speculum. The speculum will be 4" aperture and 72" focal length. This is a focal ratio of 72/4 or f18.

That is, the largest diameter of the image of a star is 2.1 seconds of arc, and if the components of a double star are seperated by a greater angle than this they will show as seperate disks or in other words the resolving power of this instrument is 2.1" of arc.
      Since the resolving power of the eye is about 4' of arc, a maximum power of 4x60/2.1 or 114x can be used without visible deterioration of the image. A 1" eyepices should give perfect seeing and a 1/2" give fair results. This is a very powerful instrument and with the 1" eyepiece giving 72x you should see clearly the craters of the moon, the rings of Saturn, the moons and cloud bands of Jupiter, star clusters, etc. It is an excellent amateur's telescope.
      A long focus mirror may be much less accurate in its surface than one of small focal ratio. In Amateur Telescope Making, page 257 (Note: This page number varies from edition to another and is invalid with the Willmann-Bell reorganization of the ATM books. Bob May), is given the departure from a parabola allowable in mirrors of various sizes and focal ratios. According to this a 4" mirror of 72" focal length may deviate from a true parabolic curve by about 750% and while the knife-edge shift for the true parabola is only .03", we are allowed an overcorrection as great as that shown by a shift of .23" or an undercorrection as great as .18"
      Since the deviation allowd in a 6" mirror of f8 is limited to about .02" under-correction, you can see the the Newtellian mirror is much simpler for the beginner to polish to a good figure.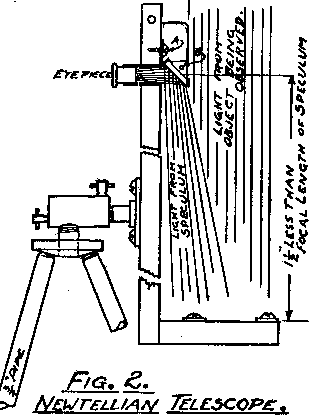
      Figure 2 shows a simple type of mount suitable for this telescope. For daylight use or where street lights are objectionable a tube may be formed by bending a long sheet of sheet metal into a trough shape and tacking it to the edges of the bar, or hoops of wire or flexible wood may be used to support a cloth tube.
      A pair of sights or a finder telescope may be mounted on hte bar. After all adjustments of the mirror and diagonal are completed, observe some prominent object and then set the sights or finder to cover this same object.
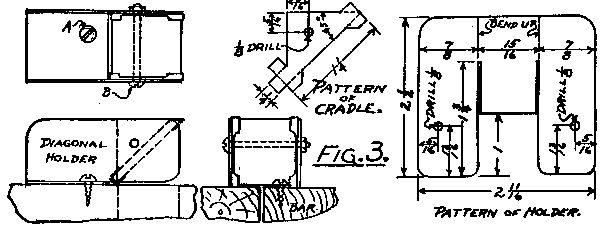
      Figure 3 shows a simple plan for holding the diagonal to give the necessary adjustments. The diagonal mirror should be 7/8" x 1 1/4". The bevel shown on one end may be ground with medium abrasive on a flat glass or metal plate. The cradles support the diagonal and are clamped with it between the two sides of the holder. In making the cradles, bend the tabs on the otted lines up on one cradle and down on the other to fit the right and left sides of the diagonal. Use thin sheet metal for the cradles, thicker for the holder.
      Adjust the diagonal by turning the diagonal around the clamp screw B and the holder around the screw A to give best definition.
      A 6" mirror of 72" focus in this type telescope will give good results if the edge of the mirror farthest from the eyepiece is covered with a crescent shaped shield. This reduces the light by about 1/4 but improves the image greatly as most of the rays producing the flare come from this area.